How to Prove Similar Triangles – Similarity Theorems

What does the word similar mean? It means something has something in common with another thing or when two things are like each other, right? We use the word similar quite casually in our daily lives, but you cannot just use the word similar when you are doing Maths. That is because the word similar has a different intent in the mathematical word. So, when you talk about similar triangles, it means a totally different thing. Here is a thing or two about triangle similarity that you should know before you dive into similarity theorems:

Similar Triangles

Let us start with what are similar triangles, what makes two triangles similar, and how to tell if triangles are similar:

Similar triangles have the same shape, but they do not have the same size. If two triangles have the same shape and size, then those triangles are identical, and they are called “congruent.”

The similar triangle reason in a pair of them is that their 3 corresponding angle pairs are always congruent while their corresponding side pairs are proportional. 

at= bu= cv

The symbol used for similar triangles is ∼. If you want to prove similar triangles like A & B, then you will write them as:

A∼B

How to Prove Triangles Are Similar?

You can use three theorems when proving triangles similar. The three similarity theorems are:

• Angle Angle (AA)

This theorem states that if two triangles have two congruent corresponding angles, you can prove their similarity. That is because if two angles are congruent, the third pair of corresponding angles has to be congruent too as angles in a triangle have a sum of 180∘.

• Side Side Side (SSS)

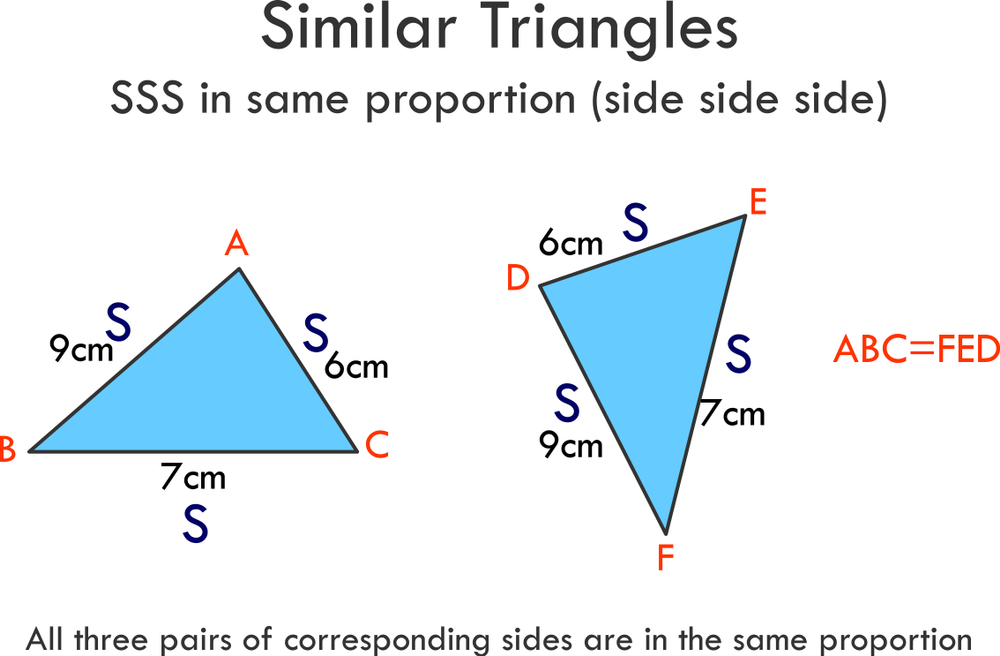
The second one among the three similar triangle proofs, the SSS theorem states that if two triangles have three corresponding sides that are proportional, you can prove their similarity. That is because, if all three corresponding sides are proportional, it’ll force the corresponding interior angles to be congruent, which means the triangles are similar.

ad=be= cf

• Side Angle Side (SAS)

If two triangles have two corresponding congruent angles, in between two pairs of proportional sides, you can prove their similarity.

ad=be

Related Read: https://www.mytutorsource.hk/blog/benefits-of-tutoring/

What Are the Properties of Similar Triangles?

• The corresponding angles have the same measurements (they are congruent).

So, for two triangles with angles P, Q, R and P’, Q’, R’, the equation will be: 

P=P’, Q=Q’, R=R’

• The corresponding sides of the triangles are all in the same proportion.

So, for two triangles with sides PQ, QR, RP, and P’Q’, Q’R’, R’P’, the right equation will be: 

PQP’Q’=QRQ’R’=RPR’P’

An Example

Theorem Question:

If any two angles of a given triangle are congruent to the corresponding two angles of another given triangle, the two triangles are similar. Prove this statement.

If: ∠A≅∠D;∠B≅∠E

Then: ABC∼DEF

Proof:

Given: ∠A≅∠D;∠B≅∠E

To Prove: ABC∼DEF

Solution:

• Since both the triangles are of different sizes, we will start this theorem by dilating the triangle ΔABC to a smaller size. However, the smaller size of the triangle has to match with the side lengths of the triangle ΔDEF. So, we will match side AB with side DE by making the scale factor KDEAB. The dilated triangle is ΔA’B’C’.
• After we have dilated the triangle, we are now aware that ΔA’B’C’∼ΔABC. The reason is that dilation is a similarity transformation.
• After the dilation:

∠A ≅ ∠A’

∠B ≅ ∠B’

Because dilations preserve the angle.

• After the dilation:

A’B’ = k•AB = DE

This will give                                              A’B’≅ DE.

• We know that ∠A ≅ ∠D, ∠B ≅ ∠E, ∠A ≅ ∠A’, and ∠B ≅ ∠B’. Now with transitive property, we get ∠A’ ≅ ∠D and ∠B’ ≅ ∠E.
• ΔA’B’C’ ≅ ΔDEF by ASA (Angle Side Angle) for two congruent triangles.
• As we know, ΔA’B’C’ ≅ ΔDEF and ΔA’B’C’ ∼ ΔABC, we now also have ΔDEF ∼ ΔABC.

Hence, proved.

Related Read: https://www.mytutorsource.hk/blog/percentage-error/

Summing It Up

These are the three main similarity theorems you need to keep in mind when your teacher asks you to prove congruence, and similar triangles. Now with this information, you can solve any question by using three diverse techniques that cover angles and sides.

Similar triangles are a very crucial part of maths. You will have to face them in one way or another in almost every grade. So, instead of shying away from them because they look difficult, pick up your pen, roll up your solves, and solve them as much as you can to become a theorem maestro!